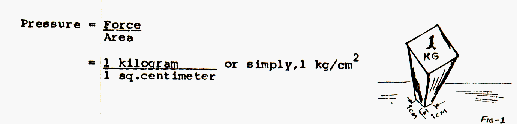
PRESSURE.
INTRODUCTION.
If an object weighs 1 kilogram and rests on one square centimeter of the earth's surface, it is exerting a pressure of 1 kilo-gram per square centimeter on the earth.
Thus, pressure is defined as Force divided by area;
Pressure = Force .
Area
= 1 kilogram or simply, 1 kg/cm2
1 sq. centimeter.
All objects have weight due to earth gravitational pull and therefore exert a pressure on the earth that can be ex-pressed in a unit of weight per unit of area (kilogram per square centimeter).
If we fill a long glass tube of 1 cm x 1 cm, with mercury to a height of 73.5 cm., then the pressure exerted by 73.5 cm of mercury column is equal to 1 kg/cm2, at 0°C.
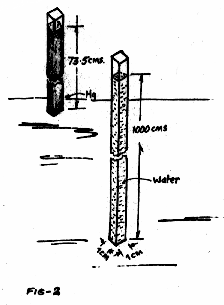
Similarly, 1000 cm of water column will also exert a pressure of 1 kg/cm2 on earth's surface at 15°C.
Thus, pressure can be expressed in millimeters of centimeters of mercury of centimeters of water are the common units of measurements.
Pressure of 1 kg/cm2 = 73. cm of mercury column at 0°C.
= 1000 cm of water column at 15°C.
Pressure, as defined above, is one of the most important of the industrial process variables. An exceedingly wide range of pressure - all the way from the ultra high vacuum where pressures as low as one-thousand of a millimeter of mercury (one micron) or greater - must be measured and controlled accurately and reliably in industrial processed. Because of this large range (10,000 psi or 703.1 kg/cm2) which is approximately ten million times as great as one micron, numerous pressure-measuring elements are required. A few of these pressure sensing elements and measuring methods are discussed in this chapter.
Scales for pressure Measurement:
There are three scales for pressure measurements,
1. Gauge Pressure Scale.
2. Absolute Pressure Scale.
3. Vacuum Scale.
The measurement of atmospheric pressure is essential to establish Gauge pressure and Vacuum pressure scale.
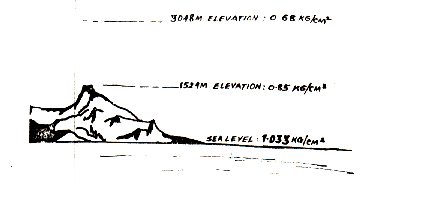
Atmospheric pressure is the pressure exerted by the air surrounding the earth. This pressure varies with altitude since the air nearer the earth is compressed by the air above.
At sea level the atmospheric pressure is 1.033 kg/cm2 (14.7 psi). At 1524 meters (5,000 ft) elevation the atmospheric pressure is 0.857 kg/cm2 (12.2 psi) and at 3048 M (10,000 ft) elevation the atmospheric pressure is 0. 682 kg/cm2 (9.7 psi).
The device used for measuring atmospheric pressure is BAROMETER.
BAROMETER: (Fig: 3)
The simplest barometer consists of a long glass tube which is uniform through out the length and sealed at one end. It is filled with mercury and then inverted and placed vertically in a pan of mercury. The mercury in the tube settles down. leaving a vacuum above it. The height of the mercury in the tube, above the level of mercury in the pan, indicates the atmospheric pressure.
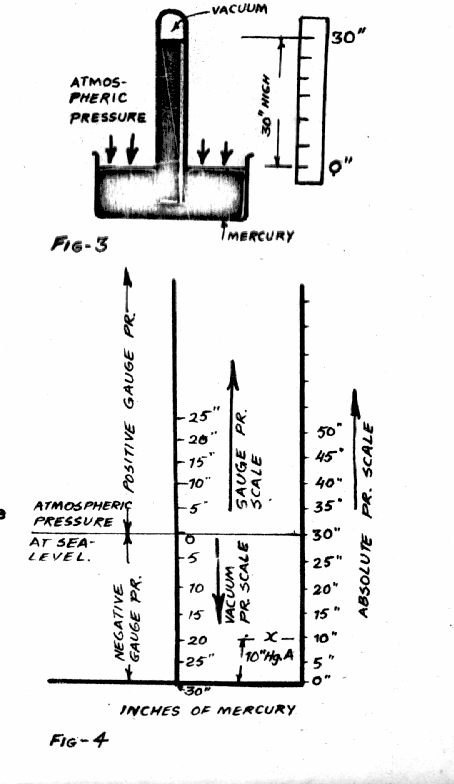
At sea level, this height of mercury column is 76 cm (30 inches)
i.e., 1.033 kg/cm2 (14.7 psi).
The zero of the Gauge pressure scale corresponds to the atmospheric pressure at that place.
The zero of the Absolute pressure scale is at the absolute pressure point. (This point is much below the atmospheric pressure, refer Fig-4).
The zero of Vacuum scale is at atmospheric pressure but its maximum point is at the absolute zero pressure point. Thus, the vacuum scale is used to indicated " negative" Gauge pressure.
From the scale chart, it can be seen that the Absolute Scale includes Vacuum scale and Gauge scale. Thus, a pressure of 10" of mercury absolute may be expressed as 'vacuum of 20" of mercury' of 'Gauge pressure of - 20" (Minus twenty inches) of mercury'.
METHODS OF MEASUREMENT OF PRESSURE
Methods of measuring pressure are classified as follows:-
1. Pressure measurement by balancing against a column of liquid of know density,
a) Simple U-tube with vertical or inclined limb.
1) Gauge Pressure measurement.
2) Absolute pressure measurement.
3) Vacuum measurement.
4) differential pressure measurement.
b) Industrial Types of Manometer.
2. Pressure measurement by balancing against a known force:-
a) Piston type pressure gauge.
b) Ring Balanced type pressure gauge.
c) Bell type pressure gauge.
3. Pressure measurement by balancing the force produced on a known area against the stress in an elastic medium:-
a. Bourdon Tubes.
1. The "C" type bourdon tube
2. The Spiral bourdon tube
3. The Helical bourdon tube.
b. Diaphragm types.
1. Still metallic diaphragm of bellows
2. Slack diaphragm and drive plate.
PRESSURE MEASUREMENT BY BALANCING AGAINST A COLUMN OF LIQUID OF KNOWN DENSITY.
Simple U-Tube:
The simplest device for measuring pressure is the Manometer and the simplest form of the manometer is the U-tube (Fig. 5) This consists of a glass tube, shaped like the letter "U" and a scale marked in centimeters (or inches) is placed in -between the two limbs.
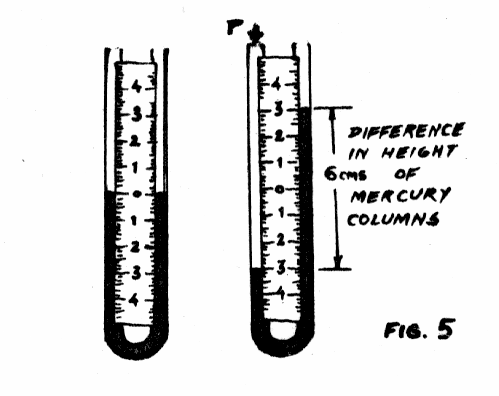
The manometric fluid (water of mercury) is poured into the tube until the level in both the limbs reaches the zero mark. With both the limbs open to the atmosphere the level of the fluid will remain at zero.
When a pressure line is connected to one limb of the manometer, the fluid in that limb will be forced down, while the fluid in the other limb will rise. By measuring the difference in the height of the fluid levels in the two limbs the pressure on the inlet line can be expressed in centimeters of fluid. For example, say the manometer causing the mercury to be lowered by 3 cms in one limb and thereby raised 3 cms in the other limb. Then the pressure in the inlet line is expressed as 6 cms of mercury, or 0.0816 kg/cm2.
Gauge Pressure Measurement:
When pressure line is connected to one limb and the other limb is kept open to the atmosphere, the height of mercury column gives the Gauge Pressure.
In Fig. 6, if h1 = 6 cm, then the Gauge pressure of the fluid is 6 cms of mercury, or
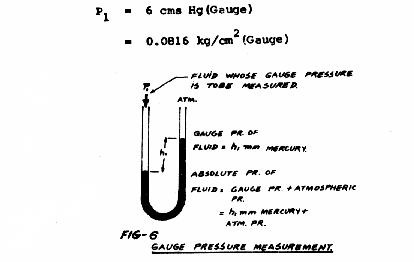
P1 = 6 cm Hg (Gauge)
= 0.0816 kg/cm2 (Gauge)
Simple U Tube in Practice:-
Consider a simple U tube containing a liquid of specific gravity pm (Fig. 7). A and B are at the same horizontal level in the liquid and the liquid at C stands at h mm above B.
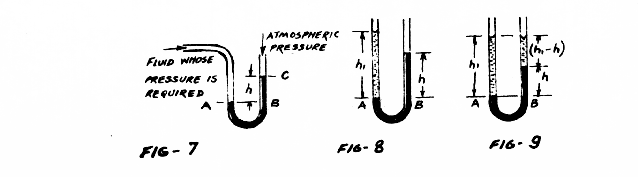
Therefore,
Pressure At A = Pressure at B
Fluid pressure at A = Atmospheric pressure + pressure due to column of liquid BC
= Atmospheric pressure + h pm mm H2O.
hpm mm H2O is the 'gauge' pressure and is written hpm mm H2O. gauge.
Wet leg correction:-
If the fluid in the left-hand limb has a density which can not be neglected in comparison with the density of the liquid in the gauge, then and allowance must be made for the pressure due to the fluid in the gauge and connecting pipes. For example, suppose the simple gauge, Fig-8, was being used to measure stream pressure, and the pipes between A and the main were filled with water whose level stands h1 mm above A. Suppose the liquid in the gauge is mercury specific gravity 13.55 at 20°C and let the gauge indicate h mm as before.
Pressure at A = Pressure at B
h1 mm H2O + Steam pressure = 13.55 h mm H2O + atmospheric pressure.
Steam pressure = (13.55 h - h1) mm H2O + atmospheric pressure.
i.e. Steam pressure = (13.55 h- h1) mm H2O gauge.
When the gauge is being used to measure the pressure differential produced by a throttling device as in steam flow measurement, both limbs of the gauge above the mercury and the pipes to the main are filled with water up to the same horizontal level, say h1 mm above A. If A is connected to the high pressure side then:
Pressure at A = Pressure at B (Figure -9)
h1 mm H2O + high pressure = (h1-h) mm H2O + 13.55 h mm H2O + low pressure.
Differential pressure = High pressure - low pressure
= { (h1 - h) + 13.55 h - h1} mm H2O
= (13.55 - 1) h mm H2O
= 12.55 h mm H2O
or in terms of mm mercury
Differential pressure = 12.55 . h mm Hg.
13.55
or in the general case when the specific gravity of the gauge liquid is Sm and of the covering liquid is Sc:
Differential pressure h Sm - Sc mm gauge liquid.
Sm
If the differential pressure is being measured by the amount the mercury in the right-hand limb rises above a fixed level, then this correction must be treated differently.
Suppose the change in level in the right-hand limp is hm mm. The provided the tube is of uniform cross-section, rise in level in the right-hand limb equals fall in level in left hand limb.

Pressure at A = Pressure at B (Fig-10)
(h1 + hm) Sc mm H2O + high pressure = { (h1-hm)Sc+2hm Sm} mm H2O+ low pressure.
Differential pressure = { (h1- hm-h1-hm) Sc+2hm Sm} mm H2O
= 2 hm (Sm-Pc) mm. H2O.
Another way of looking at his is as follows:
The greater pressure on the liquid in the left hand limb is being balance because a column of liquid of length 2 hm mm has a specific gravity of Sm instead of Sc.
Differential pressure = 2 hm (Sm - Sc) mm H2O
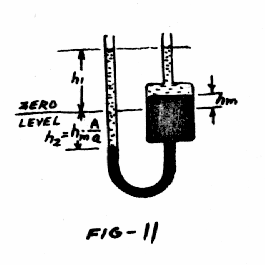
If the limbs have a different diameter, as in the case of the well type of manometer (Fig-11), then the rise in one limb will not equal the fall in the other. If the well has an area Amm, while the tube has an area amm, and the rise in the right-hand limb is hm mm as before, then since:
Loss of liquid to the left-hand limb = gain of liquid in the right hand limb,
hm A = h2a
or h2 = hm A .
a
Differential pressure = hm (1+ A ) (Sm-Sc)mm H2O.
a
Absolute Pressure Measurement:-
Consider that from one limb of the U-tube, the air above the mercury column is evacuated and then the top end of this limb is sealed. Thus, here is vacuum above mercury in this closed end limb. Now, the pressure line is connected to the other limb, the difference in mercury levels will read the Absolute pressure.
in Figure -12, if
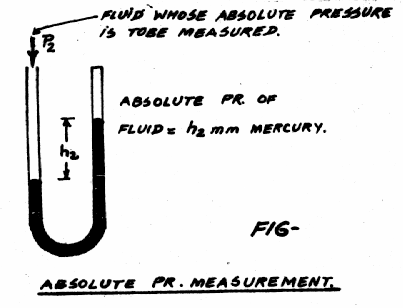
h2 = 22.5 cms then the absolute pressure of fluid.
= 220.5 cms Hg. or
P2 = 220.5 cms Hg. Abs.
= 3.0 kg/cm2 Absolute of
= 1.97 kg/cm2 Gauge + 1.03 kg/cm2 Atmp.
Now, when the pressure line is connected to the other limb, the difference in mercury levels will read the Absolute pressure.
From the above we conclude that Gauge pressure means the reading on the gauge in excess of the atmospheric pressure at the time and place of measurement. Absolute pressure is gauge pressure + atmospheric pressure at the time and place of measurement.
Example : Gauge pressure = 1.97 kg/cm2
Atms. pressure = 1.03 kg/cm2
absolute pressure = 3.00 kg/cm2
Very Small Absolute Pressure : (Fig. - 13)

The McLeod type of gauge is used for measuring very low pressures down to 2/5 x 10-2 cm of mercury. A very convenient form is shown in Figure -13.
Gas from the system whose pressure is required enters the gauge through B and fills the tubes down to the level of the mercury reservoir. The reservoir G is then raised, cutting off the gas present in the bulb H and compressing it into the capillary extension which lies along the scale S. The mercury rises faster in the left-hand limb, and may be made to above that in the closed limb. the diameter of the tube A and the closed capillary must be the same to avoid differences of pressure arising from capillary depression of the mercury in the narrow tube.
Suppose the initial pressure of the gas is p cms of mercury, the final difference in level between the mercury in A and in the capillary is h cms, the initial volume of gas in the bulb v and final volume v1, then:
By Boyle's law pv = (p + n) v1
= pv1 + hv1
p(v-v1) = hv1
p = hv .
v-v1
Now v1 is usually very small in comparison with v so this equation may be written,
P = hv1
v
It is often arranged so that when the reservoir is raised the mercury at A always rises to the level of the top of the closed capillary, which is the zero of the scale S. Then v1 = hvo, where v0 is the volume represented by one division of the scale, so that substituting in the above equation, we have
p = h2 vo
v
The scale is graduated directly according to this law. A large range of pressures is thus obtained on a relatively short scale. Pressures from 10 cms of mercury down to 1.2 cms of mercury down to 2.5 x 10-2 cms on scale S. At this extremely low end of the scale, allowance must be made for the vapour pressure of mercury.
Another method is that devised by pirani and Hall. At low pressure the quantity of heat conducted through a gas is proportional to the pressure of the gas. The amount of electrical energy required to keep a heated tungsten wire at a constant temperature, and therefore at a constant resistance, is a measure of the pressure of the gas. Thus a convenient gauge for very low pressures can be based on this principle.
Vacuum Measurement:
In the set up, as discussed earlier for Absolute pressure measurement, if we suck of draw out the air from the open limb, the mercury level in this limb will rise where as the level of mercury in the scaled limb will fall. The difference of levels in this case will indicate vacuum.
In Fig. 14, If h3 = 6 cms, then
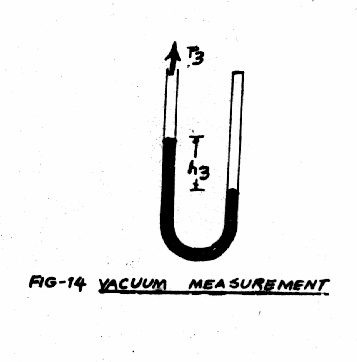
vacuum = - 6 cms of Hg. or
P3 = 0/0816 kg/cm2 Vacuum
= -0.0816 kg/cm2 Gauge.
Differential Pressure measurement:
The U-tube manometer is regarded as a differential instrument in that it responds to a difference in the pressures exerted on the liquid in limbs A and B (Fig-15)
If the Higher pressure p5 is applied to A and the lower pressure P4 to B, the liquid in A will be forced down, that in B will rise, and the action will continue until pressure p5 is balanced by the sum of pressure p4 and that due to the column of liquid h4 between the two limb levels.
Then p5 = p4 + h4 Sm
P5 - P4 = H4 Sm or
h4 = p5 - p4 : Sm being the density of the liquid Sm in the tube
The height h4 is therefore a measure of (p5 - p4), the pressure differential.
Example: Assigning some values in the above formula:
p5 + 2 kg/cm22 p4 = 1 kg/cm2 and Sm + 0.01355 kg/cm3 at 20°C
We get, Differential height h4 = (2-1)/ 0.01355 = 73.5 cms of mercury.
or h4 = 73.5 cms Hg.
Small differential Pressure:
U-tube with inclined limb: The pressure at any depth in a liquid depends only upon the density of the liquid and the depth below the surface. It is not affected by the cross-section of the vessel which contains the liquid. When it is required to measure a small difference of level this can be done by using a U tube having an inclined limb, while the other limb is reduced to a bulb as shown in Fig-16.
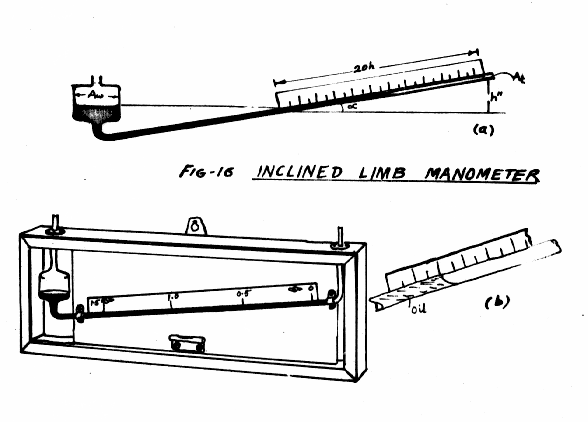
Suppose a tube is inclined at a slope of 1:20 to the horizontal, the 20 units being measured along the tube as shown in Fig-16 (a). A rise of h cms in the level of the liquid in the tube will mean that the movement of the liquid along the tube will be 20h cms. Thus, the movement for a small change in level is more easily detected than in a vertical limed manometer.
Great care must be taken, however, to keep the tube clean if the reading are to be accurate; for errors due to the changes in the force of adhesion between the liquid and the tube will be magnified in the same ratio as the movement due to change of pressure. For accurate work, allowance must also be made for the change in level in the bulb B. This can be corrected in the graduation of the scale if the sides of the bulb are parallel. If the diameter of the bulb is large in comparison with the diameter of the tube, the change of level in the bulb will be very small.
Frequently the tube is made of a plastic material, and the liquid used is a light oil so chosen that the line of the meniscus and the lines of the scale form a straight line as shown in Fig-16 (b). This enables the position of the liquid to be read more easily. Gauges of this kind usually have a range up to 4 cms water gauge, and can be read to 0.25 mm.
The relationship between differential pressure and the position of the fluid in this type of manometer (see Fig. 16a) is expressed:
Where p2 - p1 = D (1+A1/Aw) D. sin µ :
D = Density of the fluid
D. Sin µ = height of the fluid (h)
µ = angle of the inclined tube
d = length of the fluid along the inclined tube.
Industrial types of Manometer
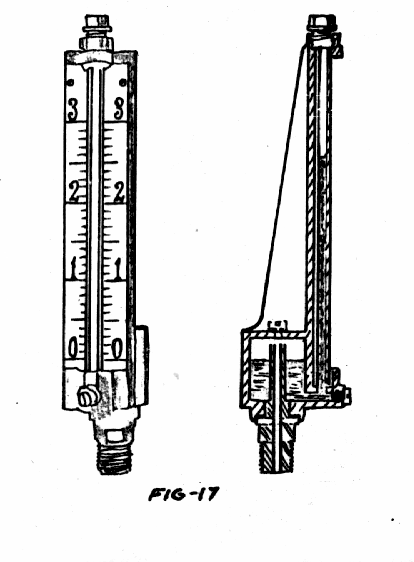
Well type:
One limb of the U tube is greatly enlarged in comparison with the other limb. When a differential pressure is applied to the manometer, the rise of the liquid on one side will not equal the fall on the other side as it does in the simple U tube. The relative sizes of the rise and fall will depend upon the diameters of the tube. Suppose a differential pressure of h mm of mercury is established between the two sides of the manometer as shown in Fig-17 (a), and suppose the diameters of tube and well are d mm and D mm respectively. Suppose the level in the will falls
xmm then: 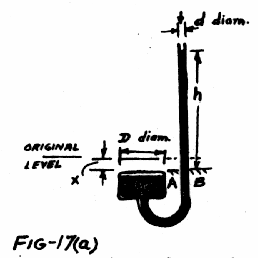
Volume of mercury leaving the well = volume entering the tube
x
x ^ D2 mm3 = (h-x) ^ d2 mm34
xD2 = h.d2
x.d2x(D2 +d2) = h.d2
x = h. d2 mm .
D2 + d2
Thus, by adjusting the diameters of tube and well, we can make z any desired fraction of h.
The relationship between differential pressure and the height of the fluid in the tube is as follows:
P2 - p1 = (1 + At / Aw) dx h ; where
d = density of the liquid
h = height of the fluid in the tube as read on the scale
(unadjusted)
At/Aw = ratio of the areas of the tube and well.
At/Aw is usually between 1/300 and 1/600. With this ration is fixed at 1/500, each scale inch is actually 1-1/500" in length or 0.998".
Mercury