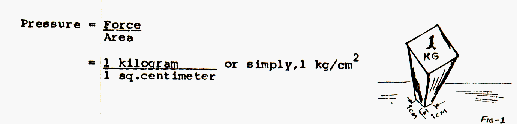
INTRODUCTION.
If an object weighs 1 kilogram and rests on one square centimeter of the earth's surface, it is exerting a pressure of 1 kilo-gram per square centimeter on the earth.
Thus, pressure is defined as Force divided by area;
All objects have weight due to earth gravitational pull and therefore exert a pressure on the earth that can be ex-pressed in a unit of weight per unit of area (kilogram per square centimeter).
If we fill a long glass tube of 1 cm x 1 cm, with mercury to a height of 73.5 cm., then the pressure exerted by 73.5 cm of mercury column is equal to 1 kg/cm2, at 0°C.
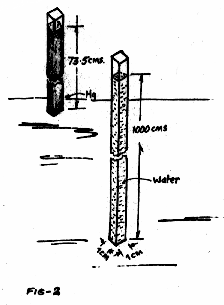
Similarly, 1000 cm of water column will also exert a pressure of 1 kg/cm2 on earth's surface at 15°C.
Thus, pressure can be expressed in millimeters of centimeters of mercury of centimeters of water are the common units of measurements.
Pressure of 1 kg/cm2 = 73. cm of mercury column at 0°C.
= 1000 cm of water column at 15°C.
Pressure, as defined above, is one of the most important of the industrial process variables. An exceedingly wide range of pressure - all the way from the ultra high vacuum where pressures as low as one-thousand of a millimeter of mercury (one micron) or greater - must be measured and controlled accurately and reliably in industrial processed. Because of this large range (10,000 psi or 703.1 kg/cm2) which is approximately ten million times as great as one micron, numerous pressure-measuring elements are required. A few of these pressure sensing elements and measuring methods are discussed in this chapter.
Scales for pressure Measurement:
There are three scales for pressure measurements,
1. Gauge Pressure Scale.
2. Absolute Pressure Scale.
3. Vacuum Scale.
The measurement of atmospheric pressure is essential to establish Gauge pressure and Vacuum pressure scale.
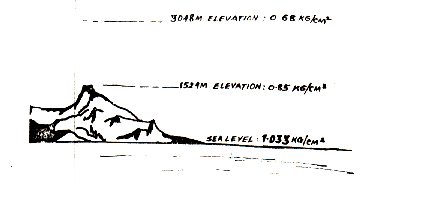
Atmospheric pressure is the pressure exerted by the air surrounding the earth. This pressure varies with altitude since the air nearer the earth is compressed by the air above.
At sea level the atmospheric pressure is 1.033 kg/cm2 (14.7 psi). At 1524 meters (5,000 ft) elevation the atmospheric pressure is 0.857 kg/cm2 (12.2 psi) and at 3048 M (10,000 ft) elevation the atmospheric pressure is 0. 682 kg/cm2 (9.7 psi).
The device used for measuring atmospheric pressure is BAROMETER.
BAROMETER: (Fig: 3)
The simplest barometer consists of a long glass tube which is uniform through out the length and sealed at one end. It is filled with mercury and then inverted and placed vertically in a pan of mercury. The mercury in the tube settles down. leaving a vacuum above it. The height of the mercury in the tube, above the level of mercury in the pan, indicates the atmospheric pressure.
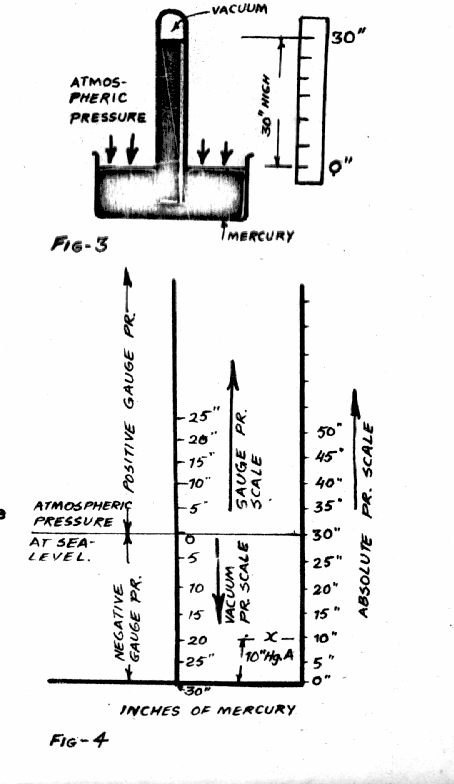
At sea level, this height of mercury column is 76 cm (30 inches)
i.e., 1.033 kg/cm2 (14.7 psi).
The zero of the Gauge pressure scale corresponds to the atmospheric pressure at that place.
The zero of the Absolute pressure scale is at the absolute pressure point. (This point is much below the atmospheric pressure, refer Fig-4).
The zero of Vacuum scale is at atmospheric pressure but its maximum point is at the absolute zero pressure point. Thus, the vacuum scale is used to indicated " negative" Gauge pressure.
From the scale chart, it can be seen that the Absolute Scale includes Vacuum scale and Gauge scale. Thus, a pressure of 10" of mercury absolute may be expressed as 'vacuum of 20" of mercury' of 'Gauge pressure of - 20" (Minus twenty inches) of mercury'.
METHODS OF MEASUREMENT OF PRESSURE
Methods of measuring pressure are classified as follows:-
1. Pressure measurement by balancing against a column of liquid of know density,
a) Simple U-tube with vertical or inclined limb.
1) Gauge Pressure measurement.
2) Absolute pressure measurement.
3) Vacuum measurement.
4) differential pressure measurement.
b) Industrial Types of Manometer.
2. Pressure measurement by balancing against a known force:-
a) Piston type pressure gauge.
b) Ring Balanced type pressure gauge.
c) Bell type pressure gauge.
3. Pressure measurement by balancing the force produced on a known area against the stress in an elastic medium:-
a. Bourdon Tubes.
1. The "C" type bourdon tube
2. The Spiral bourdon tube
3. The Helical bourdon tube.
b. Diaphragm types.
1. Still metallic diaphragm of bellows
2. Slack diaphragm and drive plate.
PRESSURE MEASUREMENT BY BALANCING AGAINST A COLUMN OF LIQUID OF KNOWN DENSITY.
Simple U-Tube:
The simplest device for measuring pressure is the Manometer and the simplest form of the manometer is the U-tube (Fig. 5) This consists of a glass tube, shaped like the letter "U" and a scale marked in centimeters (or inches) is placed in -between the two limbs.
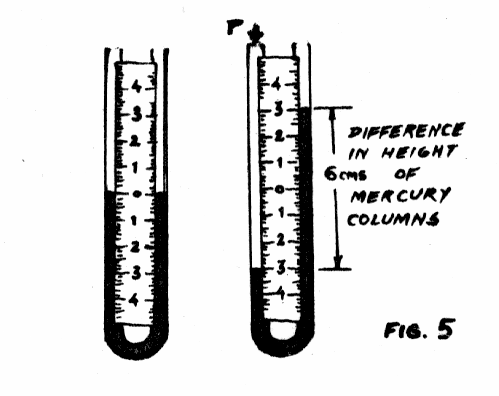
The manometric fluid (water of mercury) is poured into the tube until the level in both the limbs reaches the zero mark. With both the limbs open to the atmosphere the level of the fluid will remain at zero.
When a pressure line is connected to one limb of the manometer, the fluid in that limb will be forced down, while the fluid in the other limb will rise. By measuring the difference in the height of the fluid levels in the two limbs the pressure on the inlet line can be expressed in centimeters of fluid. For example, say the manometer causing the mercury to be lowered by 3 cms in one limb and thereby raised 3 cms in the other limb. Then the pressure in the inlet line is expressed as 6 cms of mercury, or 0.0816 kg/cm2.
Gauge Pressure Measurement:
When pressure line is connected to one limb and the other limb is kept open to the atmosphere, the height of mercury column gives the Gauge Pressure.
In Fig. 6, if h1 = 6 cm, then the Gauge pressure of the fluid is 6 cms of mercury, or
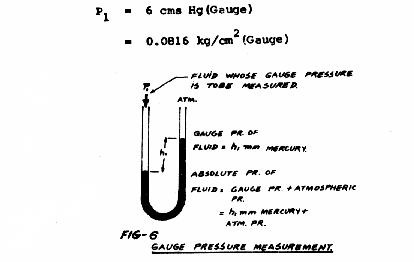
P1 = 6 cm Hg (Gauge)
= 0.0816 kg/cm2 (Gauge)
Simple U Tube in Practice:-
Consider a simple U tube containing a liquid of specific gravity pm (Fig. 7). A and B are at the same horizontal level in the liquid and the liquid at C stands at h mm above B.
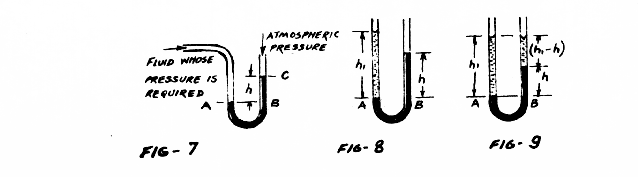
Therefore,
Pressure At A = Pressure at B
hpm mm H2O is the 'gauge' pressure and is written hpm mm H2O. gauge.
Wet leg correction:-
If the fluid in the left-hand limb has a density which can not be neglected in comparison with the density of the liquid in the gauge, then and allowance must be made for the pressure due to the fluid in the gauge and connecting pipes. For example, suppose the simple gauge, Fig-8, was being used to measure stream pressure, and the pipes between A and the main were filled with water whose level stands h1 mm above A. Suppose the liquid in the gauge is mercury specific gravity 13.55 at 20°C and let the gauge indicate h mm as before.
Pressure at A = Pressure at B
Steam pressure = (13.55 h - h1) mm H2O + atmospheric pressure.
When the gauge is being used to measure the pressure differential produced by a throttling device as in steam flow measurement, both limbs of the gauge above the mercury and the pipes to the main are filled with water up to the same horizontal level, say h1 mm above A. If A is connected to the high pressure side then:
Pressure at A = Pressure at B (Figure -9)
= { (h1 - h) + 13.55 h - h1} mm H2O
= (13.55 - 1) h mm H2O
= 12.55 h mm H2O
or in terms of mm mercury
Differential pressure = 12.55 . h mm Hg.
13.55
or in the general case when the specific gravity of the gauge liquid is Sm and of the covering liquid is Sc:
Differential pressure h Sm - Sc mm gauge liquid.
Sm
If the differential pressure is being measured by the amount the mercury in the right-hand limb rises above a fixed level, then this correction must be treated differently.
Suppose the change in level in the right-hand limp is hm mm. The provided the tube is of uniform cross-section, rise in level in the right-hand limb equals fall in level in left hand limb.

Pressure at A = Pressure at B (Fig-10)
(h1 + hm) Sc mm H2O + high pressure = { (h1-hm)Sc+2hm Sm} mm H2O+ low pressure.
Differential pressure = { (h1- hm-h1-hm) Sc+2hm Sm} mm H2O
= 2 hm (Sm-Pc) mm. H2O.
Another way of looking at his is as follows:
The greater pressure on the liquid in the left hand limb is being balance because a column of liquid of length 2 hm mm has a specific gravity of Sm instead of Sc.
Differential pressure = 2 hm (Sm - Sc) mm H2O
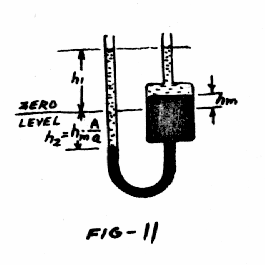
If the limbs have a different diameter, as in the case of the well type of manometer (Fig-11), then the rise in one limb will not equal the fall in the other. If the well has an area Amm, while the tube has an area amm, and the rise in the right-hand limb is hm mm as before, then since:
Loss of liquid to the left-hand limb = gain of liquid in the right hand limb,
hm A = h2a
or h2 = hm A .
a
Differential pressure = hm (1+ A ) (Sm-Sc)mm H2O.
a
Absolute Pressure Measurement:-
Consider that from one limb of the U-tube, the air above the mercury column is evacuated and then the top end of this limb is sealed. Thus, here is vacuum above mercury in this closed end limb. Now, the pressure line is connected to the other limb, the difference in mercury levels will read the Absolute pressure.
in Figure -12, if
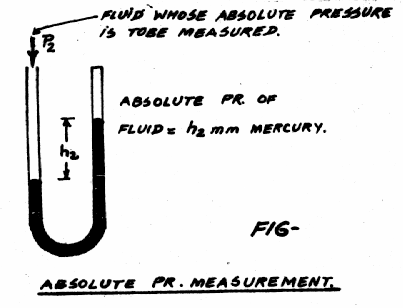
h2 = 22.5 cms then the absolute pressure of fluid.
= 220.5 cms Hg. or
P2 = 220.5 cms Hg. Abs.
= 3.0 kg/cm2 Absolute of
= 1.97 kg/cm2 Gauge + 1.03 kg/cm2 Atmp.
Now, when the pressure line is connected to the other limb, the difference in mercury levels will read the Absolute pressure.
From the above we conclude that Gauge pressure means the reading on the gauge in excess of the atmospheric pressure at the time and place of measurement. Absolute pressure is gauge pressure + atmospheric pressure at the time and place of measurement.
Example : Gauge pressure = 1.97 kg/cm2
Atms. pressure = 1.03 kg/cm2
absolute pressure = 3.00 kg/cm2
Very Small Absolute Pressure : (Fig. - 13)

The McLeod type of gauge is used for measuring very low pressures down to 2/5 x 10-2 cm of mercury. A very convenient form is shown in Figure -13.
Gas from the system whose pressure is required enters the gauge through B and fills the tubes down to the level of the mercury reservoir. The reservoir G is then raised, cutting off the gas present in the bulb H and compressing it into the capillary extension which lies along the scale S. The mercury rises faster in the left-hand limb, and may be made to above that in the closed limb. the diameter of the tube A and the closed capillary must be the same to avoid differences of pressure arising from capillary depression of the mercury in the narrow tube.
Suppose the initial pressure of the gas is p cms of mercury, the final difference in level between the mercury in A and in the capillary is h cms, the initial volume of gas in the bulb v and final volume v1, then:
By Boyle's law pv = (p + n) v1
= pv1 + hv1
p(v-v1) = hv1
p = hv .
v-v1
Now v1 is usually very small in comparison with v so this equation may be written,
P = hv1
v
It is often arranged so that when the reservoir is raised the mercury at A always rises to the level of the top of the closed capillary, which is the zero of the scale S. Then v1 = hvo, where v0 is the volume represented by one division of the scale, so that substituting in the above equation, we have
p = h2 vo
v
The scale is graduated directly according to this law. A large range of pressures is thus obtained on a relatively short scale. Pressures from 10 cms of mercury down to 1.2 cms of mercury down to 2.5 x 10-2 cms on scale S. At this extremely low end of the scale, allowance must be made for the vapour pressure of mercury.
Another method is that devised by pirani and Hall. At low pressure the quantity of heat conducted through a gas is proportional to the pressure of the gas. The amount of electrical energy required to keep a heated tungsten wire at a constant temperature, and therefore at a constant resistance, is a measure of the pressure of the gas. Thus a convenient gauge for very low pressures can be based on this principle.
Vacuum Measurement:
In the set up, as discussed earlier for Absolute pressure measurement, if we suck of draw out the air from the open limb, the mercury level in this limb will rise where as the level of mercury in the scaled limb will fall. The difference of levels in this case will indicate vacuum.
In Fig. 14, If h3 = 6 cms, then
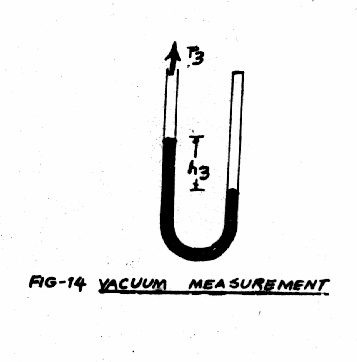
vacuum = - 6 cms of Hg. or
P3 = 0/0816 kg/cm2 Vacuum
= -0.0816 kg/cm2 Gauge.
Differential Pressure measurement:
The U-tube manometer is regarded as a differential instrument in that it responds to a difference in the pressures exerted on the liquid in limbs A and B (Fig-15)
If the Higher pressure p5 is applied to A and the lower pressure P4 to B, the liquid in A will be forced down, that in B will rise, and the action will continue until pressure p5 is balanced by the sum of pressure p4 and that due to the column of liquid h4 between the two limb levels.
Then p5 = p4 + h4 Sm
P5 - P4 = H4 Sm or
h4 = p5 - p4 : Sm being the density of the liquid Sm in the tube
The height h4 is therefore a measure of (p5 - p4), the pressure differential.
Example: Assigning some values in the above formula:
p5 + 2 kg/cm22 p4 = 1 kg/cm2 and Sm + 0.01355 kg/cm3 at 20°C
We get, Differential height h4 = (2-1)/ 0.01355 = 73.5 cms of mercury.
or h4 = 73.5 cms Hg.
Small differential Pressure:
U-tube with inclined limb: The pressure at any depth in a liquid depends only upon the density of the liquid and the depth below the surface. It is not affected by the cross-section of the vessel which contains the liquid. When it is required to measure a small difference of level this can be done by using a U tube having an inclined limb, while the other limb is reduced to a bulb as shown in Fig-16.
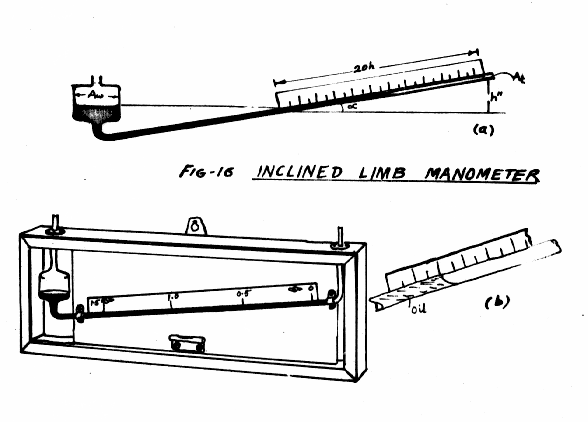
Suppose a tube is inclined at a slope of 1:20 to the horizontal, the 20 units being measured along the tube as shown in Fig-16 (a). A rise of h cms in the level of the liquid in the tube will mean that the movement of the liquid along the tube will be 20h cms. Thus, the movement for a small change in level is more easily detected than in a vertical limed manometer.
Great care must be taken, however, to keep the tube clean if the reading are to be accurate; for errors due to the changes in the force of adhesion between the liquid and the tube will be magnified in the same ratio as the movement due to change of pressure. For accurate work, allowance must also be made for the change in level in the bulb B. This can be corrected in the graduation of the scale if the sides of the bulb are parallel. If the diameter of the bulb is large in comparison with the diameter of the tube, the change of level in the bulb will be very small.
Frequently the tube is made of a plastic material, and the liquid used is a light oil so chosen that the line of the meniscus and the lines of the scale form a straight line as shown in Fig-16 (b). This enables the position of the liquid to be read more easily. Gauges of this kind usually have a range up to 4 cms water gauge, and can be read to 0.25 mm.
The relationship between differential pressure and the position of the fluid in this type of manometer (see Fig. 16a) is expressed:
Where p2 - p1 = D (1+A1/Aw) D. sin µ :
D = Density of the fluid
D. Sin µ = height of the fluid (h)
µ = angle of the inclined tube
d = length of the fluid along the inclined tube.
Industrial types of Manometer
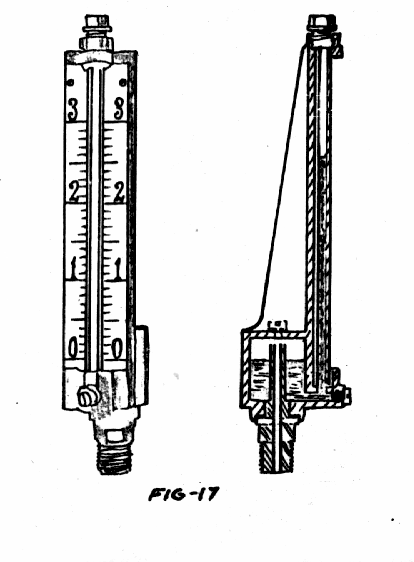
Well type:
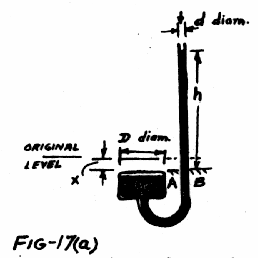
One limb of the U tube is greatly enlarged in comparison with the other limb. When a differential pressure is applied to the manometer, the rise of the liquid on one side will not equal the fall on the other side as it does in the simple U tube. The relative sizes of the rise and fall will depend upon the diameters of the tube. Suppose a differential pressure of h mm of mercury is established between the two sides of the manometer as shown in Fig-17 (a), and suppose the diameters of tube and well are d mm and D mm respectively. Suppose the level in the will falls xmm then:
Volume of mercury leaving the well = volume entering the tube
x x ^ D2 mm3 = (h-x) ^ d2 mm3
4
xD2 = h.d2 x.d2
x(D2 +d2) = h.d2
x = h. d2 mm .
D2 + d2
Thus, by adjusting the diameters of tube and well, we can make z any desired fraction of h.
The relationship between differential pressure and the height of the fluid in the tube is as follows:
P2 - p1 = (1 + At / Aw) dx h ; where
d = density of the liquid
h = height of the fluid in the tube as read on the scale
(unadjusted)
At/Aw = ratio of the areas of the tube and well.
At/Aw is usually between 1/300 and 1/600. With this ration is fixed at 1/500, each scale inch is actually 1-1/500" in length or 0.998".
Mercury float Manometer (Fig-18)
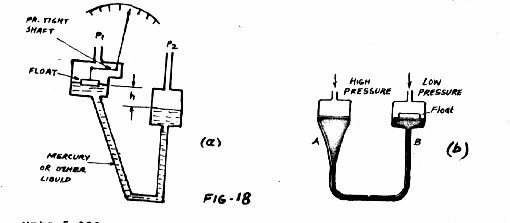
The mercury float manometer is a modification of the well type manometer. Because the buoyant force on the float is sufficient to move a recording mechanism, the mercury float manometer can be used as a differential pressure recording instrument. The manometer fluid does not have to be visible; therefore, the manometer may be made of metal permitting its use at high pressure
up to 5,00 psi. Another feature of this kind of manometer is the interchangebility of the tube columns (range tubes) to permit a change in the differential range of the instrument. The relationship between the differential pressure and height of the fluid in the will (or height of the float) is as follows:
P2 - p1 = (1+Aw/At) d.h
It can be seen that, by changing the range tubes, the ration Aw/At is changed, thus changing the range of the meter. The differential pressure ranger available with such instruments vary form 0 to 10 inches of water to 0 to 200 inches of water. Very low differential pressure ranges from 0 to 2 inches of water to 0 to 1.5 inches of water can be accommodated by substitution. oil for mercury. When such a substitution is made, special oil seals are required inside the meter body, and the instrument can be used only at low pressures.
In flow measurement, the rate of flow depends upon the square root of the differential pressure produced. By shaping the well so that its section has a profile of a certain shape it is possible to make the motion of the float hear a linear relationship to the flow; i.e., by making one limb of the shape shown in Fig-16 (b) it is possible to make the float move equal mounts for equal changes in flow. In order to do this the volume of mercury moving form chamber A to chamber B and hence the movement of the float for equal changes of head, must get steadily less. If the instrument is designed for a differential head of 100 units, and the scale is divided into 10 units, then the pointer will move 1,2, 3,4,5,6,7,8,9,10 divisions for the corresponding values 1,4,16,25,36,49,64,81 and 100 units of differential head. It is not possible, however, to have a scale which is uniformly divided over the whole range of the instrument, the beginning of the scale will be unevenly divided. This portion of the scale in a flow meter, is, however, rarely reliable, so this factor is not so important in flow indicators; but it has an important bearing on the design of mechanical integrators. Another disadvantage of the shaped limb manometer is that the quantity of filling liquid is critical, so that it can be used only with mercury and then care must be taken to ensure that the correct quantity of mercury is introduced and none lost while the instrument is in use.
Piston type (Dead Weight Tester):
Even when mercury is used as the fluid in a manometer, the pressure which may be measured by the simple type soon reaches a limit. This limit is set by the height of a column which is practicable. A convenient type of gauge which can be used for higher pressures, and in particular, for checking the elastic diaphragm or Bourdon type of gauges, is the free piston type of gauge.
In this type of instrument, the force produced on a piston of known area is measured directly by the weight it will support. In Fig-19, if the pressure acting on the piston is p kg/cm2 and
the area over which it acts is a cm2, then the force produced will be p. a kgs. If there is no friction between the piston and the walls, then the piston will support a weight W, where W = p. a kgs.
The Dead-weight Pressure Tester, shown diagramatically in Fig-19, is often used as a standard of pressure measurement. The accuracy largely depends upon the accuracy of the manufacture of the piston which must be finished to very narrow limits of error in diameter, roundness and straightens. The pistons are therefore made of hardened and tempered steel accurately ground and lapped to size. The piton is then fitted into the cylinder with the minimum of clearance and the effective diameter is presumed to be the mean of the piston and cylinder diameter. The cylinder is filled with light acid-free and resin-free mineral oil for pressure upto 8000 psi and with castor oil for higher pressures.
In order to eliminate the effects of friction, the piston is rotated while a reading is being taken. In the low pressure types (i.e. upto 8000 psi) the weights are placed directly on top of the piston, but for higher pressures this method is not suitable, since the stack of weights becomes unwieldy, and excessive frictional errors may be introduced if the weights are piled out of centre. In the high pressure models (up to 1, 20, 000 psi) an over-hand design is used, in which the piston, which may have an area or an little as 32 mm2, is fitted with a head-and-ball socket to support the weight carrier. The weight carrier consists of a platform around the bottom of a long tube. The top of the tube is domed and rests on the ball, and is therefore free to set itself so that the weight acts vertically downward through the centre of the piston, avoiding all side stresses.
The dead-weight tester is fitted with a pump for priming and as screw press for producing the pressure, the size and design of the press depending upon the highest pressure involved. The general arrangement is shown in Fig-19.
Ring Balance Type:
This type of instrument is frequently used for the measurement of low differential pressures of the order of 100 mm of water gauge. The essential portion of this instrument (shown in Fig-20) consists of hollow ring of circular section, partitioned at its upper part and partially filled with a liquid in order to form two pressure measuring chambers. The body of the ring is supported at its centre by a knife edge resting on a bearing surface or by roller-bearings or ball bearings. The ring may be of metal, such as aluminum alloy, or of a plastic moulding, the material depending upon the nature of the gas whose pressure is being measured. The nature of the gas will determine the nature of the filling medium. The quantity and the nature of this medium has no influence upon the calibration of the instrument, as it acts only as a seal. the force which operates the instrument is due to the difference between the pressures on the two sides of the partition. The cross-sectional area of the ring is therefore made large when the differential pressure to be measured is low, and less when the differential pressure is higher.

The fluids whose pressure difference is required are led into the ring by flexible connections. These are placed so that their length and movement are at the minimum. The ring is balanced by a control weight which is at its lowest point when the pressure is the same on both sides of the partition.
This type of instrument can be used for measuring at a large gauge of static pressures. The range is fixed by the nature and thickness of the material form which the ring is made and the pressure which the flexible connections will stand. For very high static pressures a half ring of steel is used with flexible connections consisting of hypodermic tubing. The fact that there is only half a ring does not alter the principle of the instrument.
The range of differential pressure for which the instrument can be used is fixed by the size of the ring and the nature and the quantity of the sealing liquid.
Although the calibration of the instrument does not depend upon the sealing liquid, the range is fixed by the maximum differential pressure which can be applied before the level of the sealing liquid falls to the bottom of the ring and allows the gas to bubble through form the high pressure to the low pressure side. This will not depend upon the size of the counter-weight, but only upon the product of the density of the liquid and the maximum permissible difference of levels. The mass of the counter weight and its position should be such that the ring rotates a convenient amount for the range of the instrument.
Bell Type:
In a bell type of pressure gauge the force produced by the difference of pressures on the outside and the inside of a bell is balanced against a weight, or against the force produced by the compression of a spring.
The instrument consists of a bell suspended with the open end downwards in a sealed chamber usually made of cast iron, containing a liquid such as oil or mercury. The liquid covers the open end of the bell and acts as a seal, so forming two chambers.
In this type of instrument (shown in Fig-21), where gravity provides the controlling force, the higher pressure is led into the inside and the lower pressure acts on the outside of the bell.

The resulting force causes the bell to rise until equilibrium is reached between the upward force and the apparent weight of the bell. As the bell rises, there is less of it immersed in the sealing liquid, so that the up thrust on it due to buoyancy is reduced. Its apparent weight will therefore increase. The thickness and density of the material form which the bell is made, its cross-sectional area, and the density of the sealing liquid, are determined by the range for which the instrument is to be used.
Since the pressure within the bell is greater than that outside, it will cause the level of the liquid on the outside of the bell to be greater than the level on the inside, as well as causing the bell to rise.
In the second type (Fig. 22) however, the bell is made of thin material and the controlling force is obtained by means of a spring. If the bell is made of thin material, the areas over which the pressures act will be equal on the inside and the outside. In this type of instrument it is usual to apply the high pressure to the outside of the bell and the low pressure to the inside.
No overload device is necessary in the bell type of instrument, for, then the differential pressure becomes great enough to force the liquid down to the level of the edge of the bell, the gas will bubble through the sealing liquid and tend to equalize the pressure until the differential returns to a measurable quantity.
The range of the instrument will be determined by the module us of elasticity of the spring and by the density of the sealing liquid. For low ranges up to a few mm Hg an organic liquid is used as a seal. For higher ranges mercury is used.
Owing to the large area of the bell this type of instrument is very useful for measuring the difference between two low static pressures.

In the pressure measuring element shown in Fig. 23, two bells inverted in a bath of oil are used. These bells are suspended from the balance beam which is carried on pivot-and socket type bearings having a very small surface contact giving small friction. The two pressures to be compared are led into the inside of the bells, and the pressure differential is indicated by the pointer which moves with the balance beam. Since the restoring force is small (being produced by the change in position of the centre of Gravity of the balance beam as it rotates) the instrument is sensitive to very small changes of pressure. Both bells are subject to the same changes of ambient temperatures, so that the instrument is unaffected by temperature changes. Changes as small as 2.5 x 10-2 mm of water gauge may be detected, and the instrument is used for controlling furnace pressure.
Models are available having differential ranges between 0-5 mm w.g. and 0-300 mm w.g at static pressures up to 145 psig.
PRESSURE MEASUREMENT BY BALANCING THE FORCE ON A KNOWN AREA AGAINST THE STRESS PRODUCED IN AN ELASTIC MEDIUM
There is a series of mechanical devices that changes shape when pressure is applied. These are called " Elastic Deformation Pressure Elements" (See Fig-24)

The table below lists these pressure elements with their lower and upper limits expressed in inches of water or in PSI.
Minimum Range Maximum Range.
Diaphragm 0" to 2" H2O 0 to 400 PSI
Bellows 0" to 5" H2O 0 to 800 PSI
Capsule 0" to 1" H2O 0 to 50 PSI
Bourdon Tube 0" to 12 PSI 0 to 100,000 PSI
Spiral 0" to 15 PSI 0 to 4,000 PSI
Helix 0" to 15 PSI 0 to 10,000 PSI
The Bourdon Tube
Because of its simplicity and versatile probably the most commonly used type of pressure gauge is that using a Bourdon tube and its modifications.
The "C" Type Bourdon Tube:
In its simplest form the Bourdon tube consists of a tube of oval section, bent in a circular arc. One end of the tube is sealed and attached by a light link work to the mechanism which operates the pointer. The other end of the tube is fixed, and is open for the application of the pressure which is to be measured. The internal pressure tends to change the section of the tube from oval to circular, and this tends to straighten out the tube. The resulting movement of the free end of the tube causes the pointer to move over the scale.
The tube is made from a variety of materials in a variety of thickness. The material chosen depends upon the nature of the fluid whose pressure is being measured and the thickness of material upon the range of measurements required. The actual dimensions of the tube used will determine the force available to drive the pointer mechanism, and this should be large enough to make any frictional force negligible.
Where no corrosion problems exist, solid drawn phosphor-bronze tubes with soft soldered or brazed joints are used for ranges of 14 - 1000 psig; solid drawn heat treated beryllium-copper tubes with brazed joints for up ranges to 5000 psig; and solid drawn ally steel tubes with screwed and welded joints for ranges 1000 to 9000 psig. When required to resist corrosion by the measured fluid, solid drawn carbon steel tubes with soft soldered or welded joints used for ranges 14 to 500 psig; drawn stainless steel tubes with welded joints for ranges 28 to 1000 psig and solid drawn "K" monel tubes with screwed and welded joints are used for ranges 1000 to 20000 psig.
The Bourdon gauge may also be used for measuring pressures less than atmospheric and can be used to measure steam pressures on boilers of stationary of locomotive engines. It is extensively used to measure water pressure, air pressure, carbon dioxide pressure and for measuring the pressure of a very large variety of other liquids and gases.

The construction of the standard concentric pressure gauge is shown in Fig. 25. The motion of the free end of the tube is communicated by means of a connecting link the lower end of the pivoted quadrant. The upper part of this quadrant consists of a toothed segment which engages with the teeth of the central pinion which rotates the pointer. The play between quadrant and pinion is taken up by a fine phosphor-bronze hair spring.
In applications where the gauge is subject to vibrations, Bakelite quadrants carried by Backbite plates are found to with stand the rough treatment better than the standard ones made of brass of phosphor bronze.
In applications such as the measurement of the pressure of a liquid delivered by a high-speed gear pump, the vibrations of the pointer may be so small that they are scarcely visible. They are, nevertheless, just as destructive to the mechanism and are therefore damped out completely by filling the case of the instrument with glycerin, which damps out these vibrations.
When Bourdon types of pressure gauges are used, the normal working pressure should not be more than 60 per cent of the maximum pressure indicated by the gauge. If this rule is adhered to, the instrument is much less liable to become inaccurate owing to changes in the material of the tube brought about by elastic fatigue. The nature of the liquid or gas whose pressure is being measured must also be considered when choosing the suitable material for the tube, as corrosion of the tube to the fluid it contains will soon alter its elastic properties and so render the instrument unreliable. If a gauge has been overloaded so that its pointer has gone off the scale, it should no longer be regarded as being accurate until re calibration has shown it to be so.
Spiral Bourdon:
The amount of movement of the free end of a Bourdon tube varies inversely as the wall-thickness and depends upon the cross-sectional form of the tube. It also varies directly with the angle subtended by the arc through which the tube is bent. In a tube having an arc of 180°, the movement of its end will be twice that of a similar tube having an arc of 90°. The movement of the free end of the tube may be increased without changing its wall-thickness, by increasing the length of the arc of the tube. When the arc through which the tube is bent reaches 360°, its length can be further increased in two ways; the tube can be made in the form of a spiral, or it can be made in the form of a helex. By increasing the number of turns in the spiral or helix an enlarged movement of the free end of the tube is obtained and the needs for a further magnifying movement avoided. In this way the need for the quadrant and pinion is eliminated, and with them the backlash which tends to occur when they become worn owing to continued use or to the presence of vibration.
The spiral form of the pressure element illustrated in Figure-26 is used for lower pressure measurement, while the helical form is used for higher pressure. The movement of the free end of the tube is transmitted to the pen arm of pointer through a flexible metal connecting strip which joins the free end to the pointer shaft. This enables the end of the spiral to move freely in a radial direction as the spiral expands. In the element shown, the spiral is made from chrome molybdenum steel tubing, all joints and closures are welded and the element heat treated to remove any stress which may have been set up in the material. This ensures uniform elastic properties in the tube. The junction between the spiral and the connecting tube is made by means of as special compression fitting.
Helical Bourdon Tube:
For higher pressures the tube is wound in the form of a helix, and this is illustrated in Fig-27. The material used for the seamless tube from which the helix is wound is determined by the nature of the fluid being metered and the range of the instrument, and follows the same practice as for "C" type Bourdon tubes. Where the nature of the fluid allows, a special bronze ally is used for ranges 14 - 600 psig; beryllium-copper for ranges between 600 and 10,000 psig; and chrome-molybdenum steel for ranges between 100 and 4000 psig, particularly in the presence of ammonia. Stainless steel elements are being increasingly used. They are used in petroleum industry where bronze is affected by corrosive compounds in the oils. In general, bronze elements are used for measuring the pressure of steam, water, air, nitrogen and similar gases.
Beryllium-copper is tough and has reliable elastic properties which make it very suitable for high pressure elements. The motion of the end of the helix is communicated to the pen in the same way as for the spiral element. The helical element is used in applications where multiple records are made on the same chart; for example, in the measurement of gas flow, where the measurement involves the knowledge of the static gas pressure and the pressure differential produced across an orifice.
DIAPHRAGM ELEMENTS
There are two types of diaphragm element design: (1) the metallic diaphragm which utilizes its own pressure-deflection characteristics and (2) the diaphragm element, generally non-metallic, opposed by a calibrated coil spring or similar elastic member. This type of diaphragm element is used only as a means of containing the pressure and exerting a force on the opposing member.
Metallic Diaphragms:
A metallic diaphragm element is primarily a device for measuring relatively low pressures. It consists of one or sever 1 capsules rigidly connected together, so that upon pressure application each capsule deflects. The total deflection is the sum of the deflections of all capsules. A capsule is composed of two diaphragms shells bonded together by soldering, brazing or welding. It may also be a single diaphragm shell bonded to a rigid plate.
A diaphragm shell is a single circular metallic disk either flat of corrugated. As generally used in industry for low-pressure measuring devices, the diaphragm shell is corrugated to improve its performance. Corrugations are formed by hydraulic or mechanical pressing of metal disks, or proper hardness. Figure-28 shows a simple metallic diaphragm element consisting of four capsules, each capsule consisting of two corrugated diaphragm shells bonded together by soldering, brazing, or welding. In this assembly, the individual capsule is connected axially with the next one and is allowed to expand unrestrained. The element can be provided with a stop for over range of under range protection.
The materials for diaphragm shells are quite extensive. Metals commonly used are trumpet brass, phosphur bronze, beryllium copper, stainless steel, Ni-Span C and Monel.
Characteristics:
The defection of a diaphragm shell is dependent upon a variety of factors :-
1. Diameter of the shell
2. Thickness of the metal
3. Shape of the corrugation
4. Number of corrugations.
5. Modulus of elasticity and
6. Pressure applied.
A diaphragm shell is normally designed so that the deflection approaches a linear relation to the pressure applied over as wide a range as possible with a minimum of hysteresis and permanent zero shift. The diaphragm shell can be designed to follow a nonlinear relationship whenever necessary.
In the design of a diaphragm, the depth, number of corrugations, and angle of formation of the diaphragm face determine the sensitivity (deflection per unit pressure) and the linearity of the diaphragm.
The sensitivity can be increase by increasing the number of convolutions and by decreasing the depth of convolution with a sacrifice of linearity. The maximum sensitivity for extremely small motions is obtained by using a flat un- corrugated diaphragm.
Application:
The element are generally used for relatively low pressure and low vacuum measurements, from ranges of 0 to 0. 2 in. water to 0 to 30 psi. Low pressure absolute-pressure gauges, draft gauges, liquid-level gauges, and differential-pressure gauges are typical types.
Simple diaphragm elements (Fig-28) are used for draft gauges, liquid level gauges and pressure and vacuum gauges.
Differential pressure gauges utilize the element, as shown in Fig-29. To measure absolute pressure, the diaphragm is evacuated and permanently sealed. The absolute pressure is applied to the outside of the element, within the sealed housing. The motion is transferred to the pointer outside the housing through a bellows seal. This device can be used for a differential pressure gauge by applying one pressure internally to the diaphragm element and using over-range stops for protection, as shown in Fig-28.
Opposing diaphragm elements can be used as shown in Fig-30, to measure absolute pressure, or if pressure is applied to both elements, differential pressure can be measured.
Non-metallic Diaphragms:
A slack or nonmetallic diaphragm frequently replaced the metallic diaphragm for extremely low pressure or vacuum. The diaphragm is coil flexible, having a very low pressure constant. The movement of the diaphragm is opposed by a spring, which determines the deflection for a given pressure.
Figure-31 shows such as diaphragm used for measuring vacuum or pressure. Figure-32 shows a unit for differential pressure. The diaphragm can be made from variety of materials, such as leather, Teflon, neoprene, polytheline, koroseal and impregnated silk.
Bellows Elements:
The bellows element is a one-piece expansible and collapsible member, axially flexible. It is formed in one continuous operation from a thin seamless tube into a deeply folded or corrugated seamless unit by either a hydraulic or mechanical method. Bellows range in size from 5/16 to 12 inches in diameter and generally consist of several folds or convolutions.
Bellows are commonly made of brass, stainless steel, phosphor bronze, monel, Everdur and beryllium copper. The type of material selected depends primarily on the corrosive conditions to which the bellows are exposed.
The Bellows can be used for applications similar to those for diaphragm elements, such as absolute-pressure gauges and differential-pressure gauges. Fig-33 represents a simple bellows pressure gauge with a deflection of the bellows unopposed. Fig. 34 shows a bellows gauge with the motion of the bellows restrained by an opposing spring, and Fig-30 shows a two-bellows differential unit or absolute-pressure unit. One of the bellows can be evacuated for use as an absolute pressure unit or can be left open to pressure to be used as a differential unit.
Figure-35 shows a single-bellows differential gauge, which measures the difference between the pressure on the outside and on the inside of the bellows. If the bellows is evacuated and then sealed off, an absolute-pressure gauge is obtained.
Figure-36 shows a double-bellows differential pressure gauge designed for low-differential pressure measurement of high static pressure. Both bellows are filled with a liquid. High pressure contracts the high-pressure bellows. The liquid is transmitted to the low-pressure bellows, mainly through the connecting passage the rate of flow being controlled by the station dampener. The span of the gauge is controlled by the span of the spring used. Motion to the pointer is obtained by means of a pressure-tight torque tube. A liquid with a low co-efficient of thermal expansion is used, such as a solution of ethylene glycol and water. A compensation bellows can be supplied to provide means for correcting reading errors that could arise because of volumetric change of the filling liquid with temperature change.
Extreme over range protection is obtained by the use of valves (See Fig-36). When the differential pressure exceeds a certain value, the valve will close and prevent any further movement of the bellows. Additional pressure can be subjected to the bellows without any damage or distortion because of the relative incompressibility of the liquid fill.
SEALS
In many pressure-measuring applications, it is desirable to prevent the process fluid from contacting or seeping into the pressure-measuring element or connecting line for either of two reasons:
1. To prevent inaccuracies in measuring-element indication due to changes in static head.
2. To protect the measuring element from corrosive fluids or congealing viscous fluids.
Protecting seals involve the use of either a protecting fluid or membrane between the process fluid and the measuring element.
Use of Protecting Liquid:
Figure-37 indicates such protection. In Fig.37b, the protecting liquid is heavier than the process fluid, with the former being connected to the measuring element by proper tubing: in Fig 37a, the protecting liquid is lighter than the process fluid, and again the protecting liquid is directly accessible to the measuring element. Between the protecting liquid and the measuring element, air is trapped which may gradually dissolve into the liquid. Hence, the liquid must be of sufficient capacity so that if the pressure element gradually fills with liquid enough of it remains in the protecting chamber.
It is necessary that the protecting liquid be insoluble in the process fluid. This method is not recommended for low-pressure measurement requiring a diaphragm element, unless the level of the protecting liquid changes relatively little so that the resultant static-head change is small compared to the measured pressure. This is possible by using a sealing container with a large cross-sectional area.
Use of Protecting Membrane:
In Fig.-38, a sealing device using a nonmetallic diaphragm, such as Neoprene R or rubber, is shown. This device is usable particularly for low-pressure measurement with diaphragm elements having large volumetric changes for given pressure changes. It can be used with all Bourdon elements. The measuring element, tubing, and diaphragm are filled with air at atmospheric pressure at some temperature reference point.
The diaphragm unit must be designed with sufficient capacity to take care of the total compressed volume change for the complete system of diaphragm, tubing, and element for the required pressure change. Also, if the ambient temperature surrounding the system should change, the diaphragm must be able to expand or contract without changing its extremely small spring constant, in order to produce a minimum change in the measuring-element deflection. Liquid- level measurement is one common application of this unit.
Volumetric Seals:
Figures 39a, b and c show sealing devices commonly called volumetric seals. These devices replace the compressible air in Figure 38 with a non-compressible liquid. The transmitting liquid should have a high boiling point, a low coefficient of expansion, a low freezing point, and be non injurious to the diaphragm and containing parts. Filling liquids include a mixture of ethylene glycol and water, glycerin and water, m-xylene, and kerosene.
Figure 39a specifically indicates a nonmetallic or metallic diaphragm rigidly held in place by clamping metallic parts. The diaphragm section, the connecting tubing, and the measuring element are filled with the transmitting liquid.
Figure 39a and b show volumetric seals where all joints of the system are completely sealed by welding or brazing. In Fig-39b a bellows is used, in Fig-39c a capsule. In both cases the spring constant of the capsule or bellows is low compared to that of the Bourdon measuring element, which is necessary for this type of design. This type is adaptable for installations where the measuring element is located at a distance of up to 200 ft from the process.
These systems are completely evacuated and then filled with the non compressible liquid. Properly designed, they will operate with less than ± 1 per cent variation in reading for ranges above 50 psi, with the sensing unit exposed to any temperature to 600°F and with ± 50°F ambient temperature change of the capillary tubing and measuring element. The upper pressure limit is only a function of the design of the housing surrounding the sensing element.
The sealed system (Figs 39b and c) has an advantage over the system with the clamped diaphragm (Fig. 39a) because the former is less susceptible to leaks because of the fact that the diaphragm or bellows seal has practically the same pressure outside and inside.
The advantages of the clamped diaphragm are:
1. Easier replacement of the diaphragm.
2. The use of many materials for diaphragms that cannot be welded or brazed, such as tantalum, lead foils, and Teflon R.